Quartz Structure

 

last modified: Friday, 06-Jan-2017 01:36:00 CET

Document status: usable, section on twinning missing

This chapter introduces the crystal structure of quartz and its relation to the symmetry and the physical properties of quartz crystals.

All renderings are based on a single data set of quartz unit cell coordinates downloaded from the now orphaned site www.molecules.org.

Fig.1.01: Projection of quartz crystal structure onto a- and c-planes     480x480, 20kb
To get an idea of quartz crystal structure and its symmetry properties, most figures show the crystal when viewed in the direction of either the a-axis or the c-axis (a and c in Fig.1.01). This corresponds to a projection of the atoms onto the a-plane and the c-plane, and not to a slice of the crystal: the atoms one sees actually lie in different planes along the a- and the c-axis.

 

Introduction - Looking through a Microscope

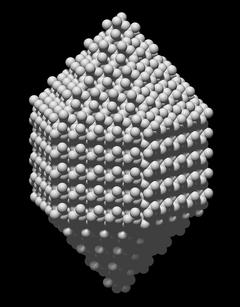
Fig.2.01: Idealized model of a quartz crystal    800x1024, 85kb
The first picture (Fig.2.01) is a computer rendering of what a tiny quartz crystal might look like in a fantasy microscope that could resolve individual atoms. This crystal would be about 7 nanometers high - 143,000 of such crystals would line up to one millimeter. In an ordinary microscope this crystal would be invisible.

Of course, this rendering is based on the assumption that atoms are just small, hard balls of identical size. Also note that while the relative positions of the atoms are correct, this is probably not an accurate model of quartz surface structure (I do not have any empirical data on that). But to explain the internal structure and the symmetry properties, it is good enough.

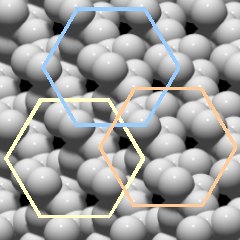
As one would expect from a crystal, one can see that there are some repeating patterns, so there is a regular structure, but as a whole it looks quite complex. The first impression is that of a very densely packed structure.

Fig.2.02: Top view of the quartz crystal model    800x800, 57kb



Fig.2.03: Hexagonal patterns

If we change the perspective to a top view of the crystal (looking down the c-axis, Fig.2.02), the relationship between the arrangement of atoms and the external shape becomes more obvious.

There are apparently hexagonal patterns that correspond to the six-sided prism of the crystal, a few examples are shown in Fig.2.03.

Another interesting feature are black holes, gaps in the structure. Since this is a top view of a three-dimensional crystal, these gaps are actually channels that run through the entire crystal, parallel to the c-axis. So the internal structure of quartz is not as tight as it first seemed.


 

The SiO4 Tetrahedron

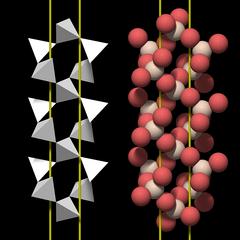
Fig.3.01:View of a quartz crystal    800x1024, 107kb
So far we have treated all atoms in the crystal structure as equal and ignored that quartz is a compound made of silicon and oxygen, SiO2.

Figure 3.01 shows a rendering of a quartz crystal similar to the one in Fig.2, with the atoms rendered as equally sized balls, but with a slightly smaller diameter. Silicon atoms are colored white and oxygen atoms are colored red.

In a real crystal, the oxygen atoms on the surface each have an additional small hydrogen atom attached. Inside an ideally grown crystal hydrogen will be completely absent, and since we are interested in the crystal structure, the hydrogen atoms have not been rendered.

This figure is nice but not very helpful for understanding the underlying structure and we need to change the perspective to gain more insight.

Fig.3.02: Cut along a c-plane     800x800, 88kb

Figure 3.02 shows a cut through the crystal along a c-plane. This is not simply a slice of the crystal with a layer of atoms, it is a top view of the crystal structure, and the atoms in it lie in different planes. So the gaps in the structure are channels that run through the entire crystal parallel to the c-axis; they have already been shown in Fig.2.02.

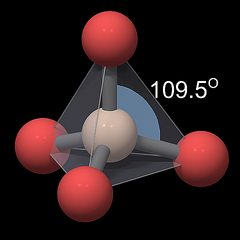
Each silicon atom is surrounded by and connected to 4 oxygen atoms, and each oxygen atom is connected to 2 silicon atoms. As explained in the chapter Chemical Properties the silicon oxygen bond is polar and covalent and not ionic. Individual silicon and oxygen atoms cannot move freely within the crystal. Thus quartz is said to have a macromolecular structure.

An ideal quartz crystal is one large molecule.

Fig.3.03: Close-up view    800x800, 81kb
Figure 3.03 is a close-up view of the same structure. Groups of silicon and oxygen are placed on a hexagonal grid. The mesh consists of SiO4-groups whose spatial orientation are different in different planes of the crystal structure.

Fig.3.04: SiO4 Group
A model of an isolated SiO4 group that seems to be the building block of quartz (Fig.3.04). The 4 oxygen atoms that surround the silicon atom form the corners of a tetrahedron. Because of its shape, the SiO4-group is called a SiO4 tetrahedron (plural: tetrahedra).

Each oxygen atom has the same distance to the silicon atom, and the distances between the oxygen atoms are also all the same.

Fig.3.05: SiO4 Tetrahedron
The tetrahedron formed by the oxygen atoms is sketched out in a ball-and-stick model in Fig.3.05 as a gray geometrical body with triangular faces. The angle of the central O-Si-O bonds is very close to the value in an ideal tetrahedron (109.5°).

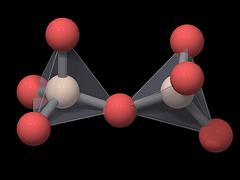
Each of the 4 oxygen atoms is linked to another silicon atom in a neighboring tetrahedron, so the SiO4 tetrahedra share their oxygen atoms and the overall formula of quartz is SiO2.

Quartz can thus be described as a network of interconnected SiO4 tetrahedra, and it is classified as a network silicate or tectosilicate.

Fig.3.06: The Si-O-Si bond forms an angle of 144°   
Although these tetrahedra are not "real", it makes sense to view the SiO4 tetrahedron as the basic unit of quartz. The SiO4 group is very rigid and can be found in almost all natural modifications of silica, and also in all silicates. What distinguishes the silica modifications is primarily the angle of the more flexible Si-O-Si bond that connects the tetrahedra. In quartz the Si-O-Si bond that links two tetrahedra is not straight (180°), but forms an angle of 144° (Fig.3.06). As a result, the crystal structure is quite complex. The chapter Chemical Properties discusses the reasons for the tetrahedral geometry and the angle of Si-O-Si bond.


 

Basic Elements of the Structure

A crystal is a solid body with a homogeneous regular internal structure. It is built up by a periodic repetition of basic elements. Those basic elements can be atoms, ions, entire molecules, or groups of them. A geometrical element that is periodically repeated to form a regular pattern is called a motif, and, in a sense, we look for motifs in the crystal structure.

The basic structural element in quartz is not a SiO2 molecule, such a molecule does not exist. And although the SiO4 tetrahedron can be considered the basic building block of quartz, it is not sufficient for characterizing quartz: there are other silica modifications with the same chemical formula that belong to different crystal systems.

So the task is to identify the characteristic element that defines the crystal structure of quartz. Or put another way: we try to find the motif that determines the pattern given in the crystal structure of quartz.

A valid motif must also reflect the chemical composition of quartz, one part silicon and two parts oxygen, so it could have a "formula" of Si2O4 or Si9O18, for example.

Fig.4.01: Projection of Si and O atoms onto the c-plane    800x800, 80kb
Figure 4.01 shows a projection of silicon and oxygen atoms onto the c-plane. The pattern looks like that in Fig.3.02 except that the atoms have been shrunken. One can identify the SiO4 groups, as each of the white silicon atoms is surrounded by 4 red oxygen atoms.

Fig.4.02: Projection of atoms and the corresponding tetrahedra    800x800, 98kb
In Fig.4.02 the tetrahedra that are formed by the four oxygen atoms in the SiO4 groups have been rendered in addition to the atoms (compare to Fig.3.05). Each oxygen atom is shared by two tetrahedra.

Fig.4.03: Network of SiO4 tetrahedra    800x800, 75kb
In the next step only the tetrahedra are rendered. Now it is very easy to identify motifs that build up the pattern. One can also see that the pattern is made of just three different tetrahedra, so the motif will contain multiples of 3 (3, 6, 9, 12,...) tetrahedra.

Fig.4.04: Motifs made of three SiO4 tetrahedra   
There are two good choices, made of three adjacent tetrahedra that are shown in Fig.4.04. The pattern in Fig.4.03 can be thought to be made of either motif a or b.

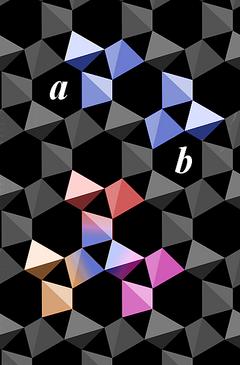
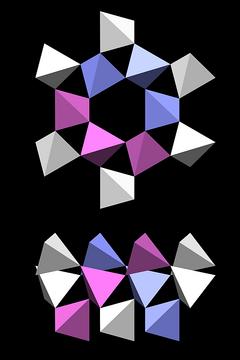
The basic structural unit of quartz is a group of three connected SiO4 tetrahedra.

Although each of the motifs a and b alone suffice to build up the entire crystal structure, it is worth mentioning that neighboring motifs share one SiO4 tetrahedron to form a group of 5 tetrahedra. As exemplified in the lower part of Fig.4.04 for motif b (multicolored with a blue center), each motif is surrounded by three motifs of the other type (orange, red, purple). That way each of the SiO4 tetrahedra is part of motif a and motif b.
(Right now it might not clear what the point about it is, but it is an important finding and we will come back to this later).

The shadows in the rendering indicate that the three SiO4 tetrahedra do not form a closed triangle - this would be an impossible geometrical figure.

Fig.4.05: Network of SiO4 tetrahedra projected onto an a-plane    800x1024, 97kb
To understand the three-dimensional shape of the motifs, we need to change perspective. Figure 4.05 is a projection of the network of tetrahedra onto the a-plane, that is, a view along the a-axis (compare to Fig.1.01). The pattern looks as if it was made of layers.

Fig.4.06: Motif made of three SiO4 tetrahedra projected onto an a-plane   
The three tetrahedra of the motif lie in three different planes along the c-axis (Fig.4.06). Repetitions of the motif along a horizontal line form the "layers" seen in Fig.4.05.

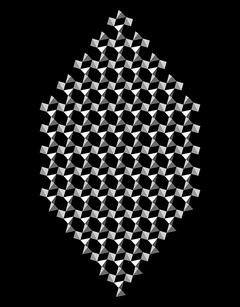
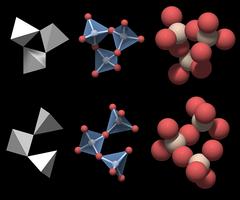
This is motif a from Fig.4.04, but it would work just as well for motif b.

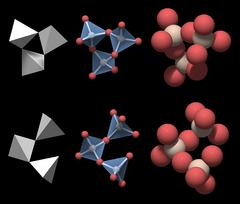
Fig.4.07: Basic structural unit of quartz    960x816, 54kb
Figure 4.07 shows different representations of the same group of three SiO4 tetrahedra, all to the same scale. The upper row shows the group from the same perspective as Fig.4.03, the lower row shows the group from the same perspective as Fig.4.05. The SiO4 tetrahedra form a little hook.

Now, there seems to be a little problem. A valid motif of the crystal structure should reflect the chemical formula of quartz, and the group in Fig.4.07 does not: if you count the atoms, its formula is Si3O10. Of course that is so because it is displayed as an isolated molecule. Inside the network of tetrahedra each oxygen is shared by two SiO4 tetrahedra, so the formula computes as (SiO4/2)3, which will give Si3O6.

The chemical formula of the basic structural unit of quartz is Si3O6.

Note: there is an alternative way of choosing a motif of the crystal structure that also works in an atomic lattice: that motif is the unit cell. Because it is defined in a more abstract way and looks confusing to the uninitiated, it is introduced later.

 

Helices

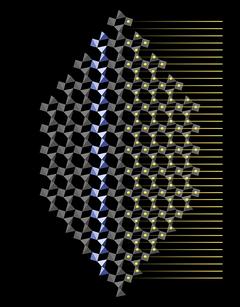
Fig.5.01: Columns of SiO4 tetrahedra and vertical distribution of silicon atoms    1024x800, 116kb
Each group of SiO4 tetrahedra is connected to two neighboring groups that lie above and below them. So the motif is repeated vertically in the pattern and forms columns. These chains of SiO4 tetrahedra run through the crystal parallel to the c-axis (blue tetrahedra in Fig.5.01). The entire crystal can be seen as a bundle of such chains.

The silicon atoms at the center of the tetrahedra occupy places on horizontal planes. In the right half of the crystal in Fig.5.01 silicon atoms (yellow dots) have been projected onto the tetrahedra pattern. The yellow horizontal lines that extend to the right demonstrate that these planes are evenly spaced along the vertical axis (or c-axis).

Fig.5.02: Helices of SiO4 tetrahedra    960x960, 91kb
Movie: helix_L.mp4, 384x384, 907kb
The chains of SiO4 tetrahedra wind around a vertical axis to form a helix. As one needs to take 3+1 steps for a full revolution (the last, 4th step is at the same angular position as the first step), this is a threefold helix. Figure 5.02 shows three different representation of these helices. Below there is a link to a H.264 movie that shows the helices revolving around a central axis.

Of course, these helices are virtual bodies - there is nothing that distinguishes the chemical bonds that connect the SiO4 tetrahedra within a particular helix from bonds to tetrahedra outside that helix. Quartz does not have a fibrous structure and it does not break more easily parallel to the c-axis. But the helices are a geometrical feature of quartz that has important implications for its symmetry.

In quartz SiO4 tetrahedra are arranged in virtual threefold helices that run parallel to the c-axis.

Fig.5.03: Left- and right-handed helices
A helix - a real-life manifestation would be a screw or a spring - has interesting symmetry properties.

First of all, a helix winds around a central axis either clockwise or counterclockwise. Clockwise and counterclockwise corresponds to being right-handed and left-handed. The left helix in Fig.5.03 is left-handed, the right one is right-handed. To determine the handedness of a helical structure (a screw for example) you place it upright in front of you and follow the helix downward with your finger. If the finger moves clockwise, the helix is right-handed. The helices shown in Fig.5.02 are left-handed.

Fig.5.04: Helix
Movie: helix_R_rot.mp4,
240x240, 61kb
Most screws are right-handed and you will note that it doesn't matter if the screw points up or down, the thread always runs clockwise. Figure 5.04 is a frame of a small H.264-movie (see link below) that demonstrates this. When the helix is rotated by 180°, it is congruent with itself - it overlaps perfectly.

This also means that you cannot bring a right-handed and a left-handed helix into congruence. To cite Wikipedia: "Handedness (or chirality) is a property of the helix, not of the perspective: a right-handed helix cannot be turned or flipped to look like a left-handed one unless it is viewed through a mirror, and vice versa."

The consequence:
A helix has no mirror symmetry.

Thus a structure that is entirely made of either a right- or a left-handed helical structure cannot show mirror symmetry. As explained in the last section (see Fig.5.02), chains of SiO4 tetrahedra form helices that run vertically through the entire crystal, and in fact quartz lacks mirror symmetry.

And that a helix is congruent with itself when rotated by 180° is in accordance with the fact that both ends of a quartz crystal show the same types of crystal faces.

Fig.5.05: Rotation of a left-handed helix Movie: helix_T3_L_rot.mp4,
384x384, 445kb
 

Fig.5.06: A left-handed helix (left), the same helix after rotation by 180° (right) and the superposition of both helices (center)    960x960, 75kb

Now it would be interesting to check if the helix presented in Fig.5.02 really behaves like the helix in Fig.5.04 when rotated. Figure 5.05 is a frame of an H.264-movie (see link below Fig.5.05) in which this helix is rotated.

Figure 5.06 shows the result: the left helix is the one already shown in Fig.5.02, but slightly rotated around the c-axis to give the best possible result. The right helix has been rotated by 180° and in the center you see the superposition of both helices.

There goes the theory, it does not work. Both helices are left-handed so the handedness is indeed preserved just as expected. But on a small scale the symmetry is broken. It does not help to shift or rotate both helices relative to each other, Fig.5.06 shows the best possible match.

The reason for the mismatch is that the outer tips of tetrahedra in the helix to the left are pointing upwards. After a 180° turn they point downwards, of course, and look a bit like leaves hanging down in the helix to the right.

A geometrical body that is not congruent with itself after a rotation by 180° is called polar. Minerals with a polar crystal structure display hemimorphism: they show different faces at both ends of the polar axis. The best examples are tourmaline and hemimorphite, the latter showing a striking difference between both ends of its elongated crystals. However, quartz crystals are not polar in their physical properties along the c-axis, and the crystallographic forms that can be found at both ends of a quartz crystal do not differ, so something must be wrong in the line of reasoning.

Fig.5.07: Top view (left) and bottom view (right) of a left-handed helix
 

Fig.5.08: Motifs made of three SiO4 tetrahedra
 

Fig.5.09: Interconnected helices    960x960, 80kb
Movie: helix_T6_L_rot.mp4,
384x384, 968kb

If we compare a top view and a bottom view of the helix (Fig.5.07), we will note that

Figure 5.08 is just a copy of Fig.4.04 and shows both motifs a and b as basic elements of the crystal structure. Some aspects have already been explained above, but so far the different inclination of the tetrahedra in the motifs has not yet been discussed.

If we pick two neighboring motifs a and b, for example the orange "type a motif" and the central multicolored "type b motif" from the lower part of Fig.5.08, and show both helices together, we get Figure 5.09 (with a tetrahedral and a atom ball model of the same helices and two yellow vertical axes; below is a link to an H.264-movie).

The central SiO4 tetrahedra in Fig.5.09 are members of two helices and the whole structure looks a bit like a chain of twisted rings. Both helices show the same handedness.

Although they contain the same types of tetrahedra, the two helices differ in polarity (compare to Fig.5.06). Whether a tetrahedron points downwards or upwards depends on the position of the vertical axis that defines the helix.

So we can summarize:

Each SiO4 tetrahedron is member of two virtual helices of the same handedness, but of opposite polarity.

Because each SiO4 tetrahedron is member of two helices of opposite polarity, this structure is not polar as a whole.


Fig.5.10: Rotating a quartz slice around an a-axis.    Movie: slice_c_plane_rot.mp4,
384x384, 634kb
If we rotate a slice of quartz that is cut parallel to the c-plane, we would expect the pattern to remain the same. This is shown in Fig.5.10, and in a H.264 movie with a link below. The upper part is a frame of the movie with the right part of the slice revolving around an a-axis. You can see that the slice is not just a thin layer of atoms or tetrahedra.

In the lower half you see the result of a rotation by 180°. The pattern in the right part of the slice still shows the same structure as the left part.

The polarity of the individual helices has no effect on a large scale, because the total polarity is balanced.

You will note that the pattern has been shifted, and both halves of the slice don't match anymore. This is an indication for a lack of mirror symmetry of the entire crystal structure, just as each helix by itself lacks mirror symmetry.

Fig.5.11: Handedness and polarity of helices    480x420, 43kb
Figure 5.11 summarizes the findings we got so far. Groups of three adjacent SiO4 tetrahedra are stacked vertically to form small helices that run parallel to the c-axis. As indicated by the yellow arrows, all helices have the same handedness (in this case all are left-handed), but differ in polarity (indicated by the arrows either pointing upwards or downwards). Each SiO4 tetrahedron is member of two helices of opposite polarity.

It is remarkable that a three-dimensional pattern built up by a motif that is both handed and polar does show handedness but no polarity. I leave that to the mathematicians to explain. However, I should stress that the helix is just a virtual body that helps to visualize an inherent symmetry property of the crystal structure of quartz, its handedness.

 

Large Channels and Double Helices

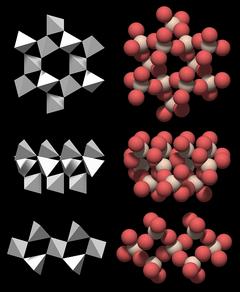
A very distinctive feature of quartz crystal structure is the presence of channels that run through the entire crystal parallel to the c-axis.

These channels are an important element of the crystal structure because they are wide enough to take up small cations.

Fig.6.01: Large channel    960x1168, 103kb
In Figure 6.01 the SiO4 tetrahedra around a channel are projected onto the c-plane (top), the m-plane[1] (middle) and the a-plane (bottom). The central channel looks like a distorted hexagon that is enclosed six tetrahedra. It is surrounded by six motifs[2] (three of type a and three of type b).

The structure is a complete ring that is roughly hexagonal. If one could take it out of the crystal, it would not fall apart.

Fig.6.02: Six helices form the wall of a central channel    576x576, 31kb



Fig.6.03: Arcs at opposite sides of the channel    576x864, 39kb

Figure 6.02 shows a top view of the ring. The central gap is surrounded by six threefold helices. All of them show the same handedness, in this case they all are left-handed, as indicated by the outer yellow arrows. If you compare with Figs.5.03, 5.06, and 5.09, you will see that the arrows point in the downward direction of each helix, so if the helix was a staircase and you were following the arrow, you would be going downstairs.

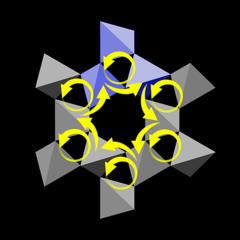
Let us look at the topmost motif in the ring, marked blue, and the two tetrahedra that are part of the wall of the central channel. Because the helix is left-handed, the right tetrahedron is lower than the left one, and this downward step is indicated by a short yellow arrow.

The same is true for all motifs. So if we pick one of the six central tetrahedra and move to the next one in clockwise direction, then to the next one and so on, we will describe a circle with a downward motion. In other words:

The tetrahedra that surround the large channels form a sixfold helix.

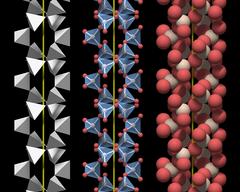
Now the ring structure shown in Fig.6.01 is only three tetrahedra high, so a sixfold helix around the central channel cannot be complete. Instead, the tetrahedra form two independent arcs made of three SiO4 tetrahedra at opposite sides of the channel, colored blue and purple in Figure 6.03.

At the bottom of Fig.6.02 we see that both arcs are winding down clockwise.

Fig.6.04: Double helix    880x800, 66kb
Movie: double_helix_tetra.mp4
384x320, 746kb
If we stack the rings, the arcs will link to form a double helix (Fig.6.04, with a link to an H.264 movie below)). The left model only shows the inner tetrahedra that make up the double helix, whereas the right model shows all tetrahedra of the ring.

This double helix is made of two sixfold helices that wind around each other with the same handedness (in this case both are right-handed). They are independent, that is, they do not directly touch each other. Both helices are connected by the outer gray tetrahedra.

The walls of the central channels are made of a sixfold double helix of tetrahedra.

Fig.6.05: Threefold and sixfold helices    920x1200, 110kb
Movie: helix_3+6.mp4
384x320, 766kb
Figure 6.05 depicts the relationship between the threefold and sixfold helices. Three of the six threefold helices that surround the central channel are marked red, orange and yellow in the left model. In the right models those tetrahedra that belong to the double helix have been marked blue and purple. The remaining gray tetrahedra that belong to the other type of motif have been omitted for clarity in the side views of both models.

Fig.6.06: Double Helix    480x420, 56kb
We can complete the image in Fig.5.11 and put double arrows into the large channels that indicate the handedness of the double helix. The result is seen in Fig.6.06 and can be summarized as:

The sixfold double helix and the threefold helices are of opposite handedness.

And another fact that can be found when inspecting Fig.6.06:

Each SiO4 tetrahedron is member of 2 threefold and 2 sixfold helices.

Fig.6.07: Double Helix    1200x960, 152kb
Movie: double_helix.mp4,
480x384, 1.7MB
Figure 6.07 shows three representations of the same double helix, similar to the models of the threefold helix in Fig.5.02. The billiard ball model to the right reminds one of images of a much more famous and important double helix: the DNA double helix, the substance that carries the genetic information in chromosomes. There is an interesting difference between DNA double helix and the quartz double helix that can also help to understand a symmetry property of the double helix: in DNA, those parts of the molecule that carry the genetic code point to the center of the helix. If DNA was tailored like quartz, its structure would be different[3]: 

natural DNA         Quartz Design
 5'      3'         5'    3'
 PR-A::T-RP         PR-A::PR-A
 PR-G::C-RP         PR-G::PR-G
 PR-C::G-RP         PR-C::PR-C
 PR-C::G-RP         PR-C::PR-C
 PR-A::T-RP         PR-A::PR-A
 PR-T::A-RP         PR-T::PR-T
 3'      5'         3'    5'
In a quartz-type DNA the members of individual base pairs would simply be two identical molecules, and they would not face each other, but point in the same direction. In quartz, equivalent sides of a pair of tetrahedra do not face each other because they both assume the same orientation in space and "point" in the same direction. And of course the tetrahedra do not attract each other as the members of a base pair do.


 

Rotational and Mirror Symmetry

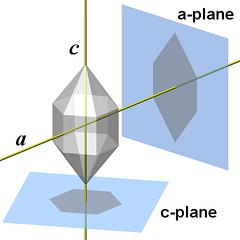
All symmetry properties of quartz can be deduced from the figures and findings that have been presented above. But to explicitly discuss them along with the other properties of the structure would have complicated the already long-winded presentation. So I start all over again and follow an independent approach.

To get a better idea of the rotational and mirror symmetry of quartz crystal structure, we project the atoms onto the a- and the c-plane (compare to Fig.1.01). We treat all atoms equal and for now do not distinguish silicon and oxygen atoms.

Fig.7.01: Projection of quartz crystal structure onto the c-plane    800x800, 77kb

Fig.7.02: Channels in quartz structure    480x400, 38kb

We begin with a projection onto the c-plane, which is the same perspective as the top view in Fig.2.01. The result is a beautiful pattern that looks much like an oriental ornament (Fig.7.01). Again, this is not a slice of the crystal: the atoms we see actually lie in different planes along the c-axis.

We see large channels that correspond to the small gaps in Fig.2, and twice as many smaller channels (see Fig.7.02).

This is obviously not a hexagonal structure, it is trigonal and has a threefold rotational symmetry. If you take that pattern and rotate it by 120°, it will match, but if you just rotate it by 60°, it will not.

At first it seems that this is only so because the pattern is made of triangular groups of three atoms, but that's not all that is to it.

Fig.7.03: Projection of Si atoms onto the c-plane    800x800, 30kb
If we take away all oxygen atoms, we get a pattern made of silicon atoms as shown in Fig.7.03. At first it looks as if the structure had a higher symmetry now and was hexagonal, but in fact the structure is distorted: the outlines of the large channels are not perfect hexagons and the triangles that surround the smaller channels appear to be slightly twisted. The deviation from the hexagonal symmetry is subtle and you have to a look at the large version of the image to see it.

Fig.7.04: Apparent mirror planes    400x400, 48kb
 
The pattern in Fig.7.01 not only shows rotational symmetry, it also appears to be mirror symmetric. Figure 7.04 depicts a few possible mirror planes that run through the pattern parallel to the a-axes.

Quartz does not show mirror symmetry, however, and the mirror symmetry of the pattern is only an apparent one - so far we have disregarded the fact that the structure is three-dimensional.

Fig.7.05: Projection of atoms onto the a-plane    800x1024, 84kb
Figure 7.05 shows the projection of the crystal structure onto an a-plane, so it is a view along one of the a-axes (see Fig.1.01). The pattern looks completely different now. If the a-axes truly were mirror planes, as suggested by Fig.7.04, we should now also see a pattern that is mirror symmetric, but we don't. So quartz structure as a whole lacks mirror symmetry.

If this pattern is rotated by 180° it will look the same, it shows twofold rotational symmetry. This sounds rather trivial, but remember that this is not true for the trigonal pattern in Fig.101, it has to be rotated by 120° to match, and a 180° rotation will not work.

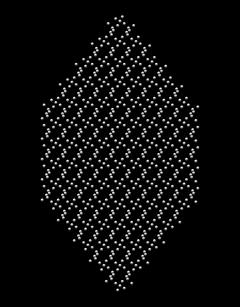
Fig.7.06: Projection of atoms onto another a-plane    800x1024, 86kb
This is just another view along along another a-axis, the pattern looks the same, except that it is flipped (Fig.7.06).


We can summarize that quartz is trigonal and shows

  • a threefold rotational symmetry around the c-axis
  • a twofold rotational symmetry around the a-axes
  • no mirror symmetry

    • It is accordingly given a Hermann-Mauguin symbol of 32 (read "three-two").

    The pattern seen when viewing down the c-axis (Fig.7.01) is very different from the pattern seen when viewing along an a-axis (Figs.7.05 and 706). Such a structure will likely react differently to forces acting from different directions, it is anisotropic. This anisotropy of quartz in fact has important technical implications.


     

    Handedness

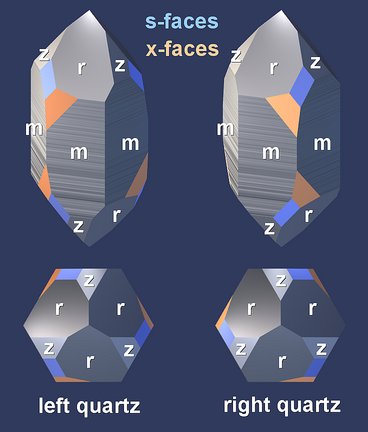
    left_right_quartz_x+s.jpg
    Fig.8.01: s- and x-faces on left- and right-handed crystals
    Ideal quartz crystals are either left- or right-handed, as determined by the position of certain crystal faces. Figure 8.01 shows s-faces (tinted blue) and x-faces (tinted orange) on both left- and right-handed quartzes and their relative position to r-, z-, and m-faces. Left and right quartz are mirror images of each other.

    The basis of this handedness in crystal morphology is the handedness of the internal structure, as found in the handedness of its elementary unit (the group of three SiO4 tetrahedra) and in the handedness of the virtual helices that are made up by these groups.

    All figures that have been presented so far show renderings of a right-handed quartz structure. We will have a look at the key elements of quartz crystal structure to see how they change with handedness.


    Fig.8.02: Right-handed quartz    960x800, 55kb
     

    Fig.8.03: Left-handed quartz    960x800, 54kb

    Figures 8.02 and 8.03 show top and side views of different representations of the elementary unit made of three SiO4 tetrahedra in right- and left-handed quartz.

    The differences are subtle. The handedness can be determined by checking if the hook described by the three tetrahedra runs in a clockwise or counter-clockwise direction.

    Although the outer shapes of the models are very similar, it is not possible to bring them into congruence, no matter how you rotate them. For example, the tetrahedra in the upper left top views of both figures point in different directions.

    Fig.8.04: Left- and right-handed threefold helices    800x800, 62kb
    Movie: L_R_helices.mp4,
    384x384, 406kb
    The left- and right-handed quartz crystals shown in Figure 8.01 are mirror images of each other. This relationship can also be found in the crystal structure and can be demonstrated by placing two helices of opposite handedness next to each other. Figure 8.04 shows left- and right-handed forms of the threefold helix. Under the figure there is a link to an H.264 movie that shows both helices rotating in opposite directions.

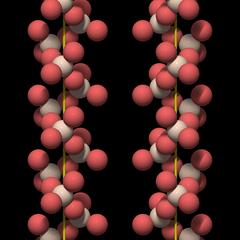
    The handedness of quartz crystal structure is not only expressed in the geometry of quartz crystals, it also plays a role in its optic properties. Quartz is an optically active substance: it rotates the polarization plane of light that passes through it along its c-axis (see the chapter Physical Properties for an introduction).

    The relationship between the handedness of the threefold virtual helices, the morphology and the physical properties is straightforward. The following table lists morphological, structural and optical features of left- and right-handed quartz.

      Position of
    s- and x-Faces    		 Handedness of
    Threefold Helix    		 Handedness of
    Sixfold Helix    		 Rotation of
    Polarization Plane
     Left Quartz left right
    (clockwise)    		 left
    (counter-clockwise)    		 left
    (counter-clockwise)
     Right Quartz right left
    (counter-clockwise)    		 right
    (clockwise)    		 right
    (clockwise)



     

    Unit Cell of Quartz

    Fig.10.01: Basic structural unit of quartz    960x816, 54kb
    In all crystals (not only in quartz) molecules and atoms are arranged in a regular grid. The shape of the crystal is primarily based on the specific geometrical characteristics of the crystal lattice that is made of periodically repeated motifs.

    So far we have identified a few possible motifs of quartz crystal structure, one of them is presented again in Fig.10.01. These motifs could be used to build up the entire three dimensional pattern simply by periodically repeating them in space. But what has this strangely formed hook made of three SiO4 tetrahedra to do with the six-sided prisms of a quartz crystal?

    It is obviously very difficult to grasp the relationship between the geometry of the motif and the geometry of the crystal.


    Crystallographers follow a different approach. They describe the crystal structure quantitatively and look for measures that help to relate the structure to the outer shape of the crystal.
    They work with the unit cell concept:

    The benefit of this approach is that the positions of the atoms in the crystal structure, the geometry of crystallographic forms and the position of the corresponding crystal faces can now all be defined with respect to a single coordinate system.

    This is all a bit abstract, but it becomes quite clear once we look at a "real" unit cell. Since we already know a possible motif of quartz crystal structure, it is fairly easy to figure out the shape and the dimensions of its unit cell.

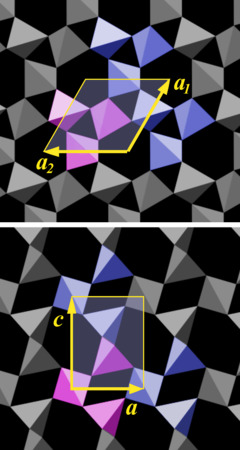
    Fig.10.02: Determining unit cell dimensions in quartz    400x768, 61kb
    We start with the "tetrahedra model" of the crystal structure because motifs can be identified in it easily.

    We pick motif a, marked as a purple group of tetrahedra in Fig.10.02, and measure how its copy must be shifted to built up the pattern of the crystal structure. One can just select some arbitrary element within the motif, for example the joint between two tetrahedra (the position of an oxygen atom) and determine the direction and the distance to the nearest equivalent point in the crystal lattice. This works with any motif of the structure, of course.

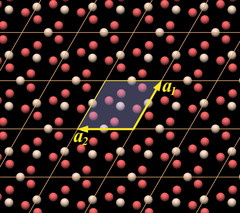
    The upper part of Fig.10.02 is a view of the c-plane, a close-up of what is shown in Fig.4.03. There are essentially three different directions along which the motif is tiled, indicated by three yellow arrows, designated a1 and a2. These arrows all have the same length. a3 is omitted, because it is unnecessary for building up the pattern[5]. The arrows a1 and a2 define a parallelogram, the geometric figure we look for. A beginner will likely pick a triangle or a hexagon, as the motif itself has a roughly triangular shape and can be repeated in six directions. But only a parallelogram can be tiled to cover a plane without leaving gaps[6].

    What is still missing is the vertical dimension of the unit cell. In the lower part of Fig.10.02 we look at the m-face of a crystal, parallel to an a-axis. Here the two arrows, designated c and a1 (the one already shown in the upper part of the figure), define another parallelogram, in this case a rectangle. Arrow c is longer than arrow a1 (and in turn a2 and a3), the ratio of their lengths is:

      c  :  a  =  1.10013  :  1

    The absolute length of the axes is:
      a   0.49133 nm or 4.9133 Å
      c   0.54053 nm or 5.4053 Å

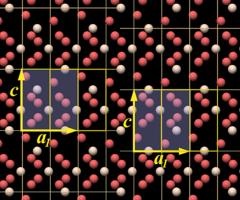
    Fig.10.03: Unit cell candidates    576x512, 59kb
    Figure 10.03 shows the unit cell in a projection of the atoms positions onto the c-plane (similar to what has been shown in Fig.4.01; silicon marked white, oxygen marked red).

    So far we have only determined the dimension of the unit cell, but not its position relative to the crystal lattice, and accordingly there are several candidates for unit cells shown as examples at arbitrary positions in the rendering. The topmost one with the letters at the arrows is at the same position as the one in Fig.10.02. They all work in the sense that by tiling them you get the complete crystal structure.

    Fig.10.04: Quartz unit cell and point lattice    576x512, 69kb
    The horizontal position of the unit cell of quartz is defined as shown in Fig.10.04. One corner of a unit cell is supposed to be placed at a position of high symmetry, and the center of the large channels is such a spot. The additional lines that run through the structure form the point lattice that is defined by the edges of the unit cell.

    Fig.10.05: Two common quartz unit cell positions along the c-axis    576x480, 63kb
    While there is general consensus about the horizontal position of the unit cell of quartz, there are two "schools" that place the unit cell of quartz at different positions along the c-axis, or its vertical position (Fig.10.05).

    I will consider the choice between the two possibilities a merely academic question and will use the left unit cell model[7]. I mention this only because you will find different unit cell models in the literature and in the Internet (to those of you that are acquainted with crystallographic terminology: the left one is a face-centered, the right one is a body-centered unit cell).

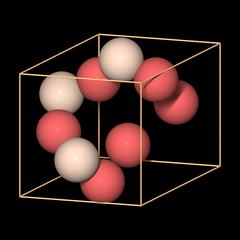
    Fig.10.06: Unit cell of quartz    800x800, 42kb
    Figure 10.06 shows a billiard ball model of the quartz unit cell. The atoms enclosed in the unit cell form another motif of the crystal lattice. The chemical formula of that group of atoms is Si3O6, just as the other motifs that have been introduced before, and reflects the overall formula of quartz, SiO2.

    Unit cell data are typically given like this:

    a  4.913Å
    c  5.405Å
    Z  3

    We know a and c already, and Z is the number of formula units in the unit cell: Si3O6 equals 3 × SiO2.

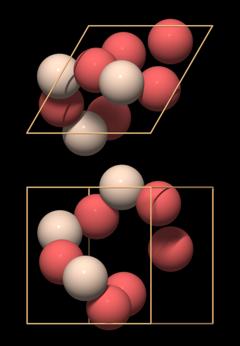
    Fig.10.07: Unit cell of quartz, top and side view    800x1152, 61kb
    The unit cell of quartz is a rhomb. Figure 10.07 shows a top and a side view of the unit cell.

    We can also recognize a key element of quartz crystal structure in it: in the lower left half there is a chain made of oxygen and silicon atoms that forms a hook. It is a part of a threefold helix, which in this case is left-handed.

    Other than that, the arrangement of the atoms looks very strange and enigmatic. In particular, what is missing from it are SiO4 tetrahedra that are the essential building blocks of quartz and silicates. By placing the unit cell at that position, the tetrahedra have been ripped apart.

    This model of the unit cell emphasizes the correct representation of the chemical formula.

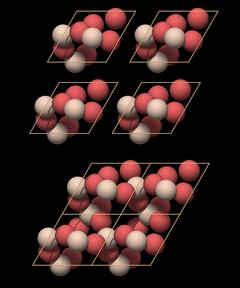
    Fig.10.08: Four adjacent unit cells    800x960, 77kb
    The unit cell presented has an almost bizarre look, so to assure oneself that it is a valid unit cell and to visualize the idea behind the unit cell concept, Fig.10.08 demonstrates how unit cells can be stacked and what the result looks like.

    On top there are 4 isolated unit cells that are simply pushed together at the bottom. This assemblage already shows all structural elements of quartz:

    Note that since four different corners of the unit cell meet in the central channel it also means that all fractions of the double helix are contained in a single unit cell.

    A single unit cell does enclose all essential structural elements of the quartz crystal lattice.


    Now you will perhaps say, "So what? You have just extracted that unit cell from the structure, of course it works!" The point is that one just needs to know the position of the atoms in unit cell coordinates and the unit cell dimensions to construct the entire crystal structure with all its features. Which, of course, is eminently practical if you want to communicate and compare data.


    Fig.10.09: Unit cell (blue) and hexagonal crystallographic axes projected into a quartz crystal.
    The unit cell of quartz is a rhomb. This rhomb corresponds to a hexagonal unit cell. The relationship between the rhomb-shaped unit cell and the hexagon, and the position of the unit cell to the quartz crystal is shown in Fig.10.09.

    The vertical planes of the unit cell correspond to the hexagonal prism faces, the m-faces. The upper and lower face of the unit cell lie parallel to the c-plane, however, the corresponding c-face is extremely rare and apparently only found on crystals that are corroded.


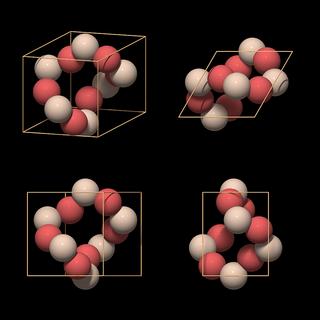
    Fig.10.10: Ring type unit cell    960x960, 65kb
    The unit cell can also be drawn in a different way, as shown in Fig.10.10 from different perspectives. Three silicon atoms are added, and now there is one silicon atom on each of the unit cell walls. Silicon atoms at opposite faces of the unit cell are at the same relative position on that face. The six silicon atoms are shared between neighboring unit cells, so the formula of the unit cell remains Si3O6.

    The atoms form a twisted ring in which each silicon atom occupies one of the faces of the rhomb. Such a unit cell type is called face-centered, because the lattice elements are placed within the faces of the unit cell[8]. In the top view we can see what is causing the twist in the ring: the unit cell encloses two neighboring threefold helices. The twisted rings have already been mentioned and can be recognized in Fig.5.09.

    Fig.10.11: Unit cell with SiO4 group    960x960, 86kb
    The most common way of presenting the unit cell of quartz is shown in Fig.10.11. Strictly spoken, this doesn't count as a valid unit cell, but since the unit cell frame is shown, one can still tell its true dimensions.

    This model is preferred because it shows the most important structural element of quartz: the SiO4 tetrahedron. When we compare it with the "ring-type" unit cell in Fig.10.10, we see that each silicon atom has been replaced by a complete SiO4 tetrahedron.

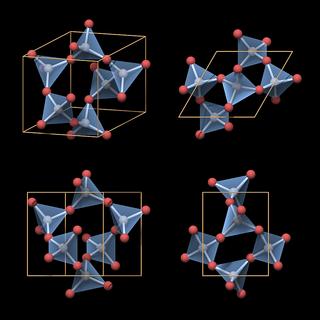
    Fig.10.12: Unit cell with emphasis on tetrahedra    960x960, 83kb
    This becomes more obvious in Fig.10.12, which emphasizes the tetrahedral units. What has been said about the silicon atoms in the ring type unit cell is also true for the tetrahedra here: tetrahedra at opposite faces of the unit cell lie at the same relative position on that face. In a sense, with respect to SiO4 tetrahedra, this is a face-centered unit cell. The tetrahedra at opposite faces assume the same orientation in space, but this is to be expected: the relative positions of atoms within the unit cell are invariant to translation - just look at Fig.10.08 to see why.

    Also note the lack of mirror symmetry, which is in agreement with the overall symmetry of the crystal structure. The top view looks mirror symmetric at a first glimpse, but if you take into account the three-dimensional structure you can see it is not.


    Fig.10.13: Four adjacent tetrahedral-type unit cells     1024x768, 60kb
    Figure 10.13 is probably the most common representation of quartz structure and if you browse the Internet or read textbooks, you will find many similar figures. It is simply an assemblage of four "tetrahedra type" unit cells.


    Fig.10.14: Some eye candy    1600x1200, 230kb
    Finally, I'd like to conclude this chapter with some eye candy. This is all about aesthetics, no need for an explanation. It would have been a sin to put a small version of it online, so it is 1600x1200.


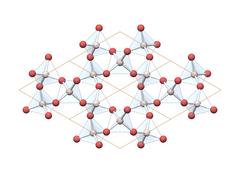
    Done.
    Time for an Aspirin ;-)


     

    Further Information, Literature, Links

    The data of quartz unit cell coordinates for rendering these images have been downloaded from www.molecules.org.


    The page The Crystal Structure of Quartz (SiO2) is a good introduction into quartz crystal structure using different views (tetrahedra, ball-and-stick, etc.). Also gives a nice demonstration of the unit cell concept applied to quartz.

    An animated gif showing the transition from low to high quartz can be found on Using XtalDraw to Make Animated GIF's: The High-Temperature Phase Transition of Quartz

    Crystallographic data can be retrieved from the American Mineralogist Crystal Structure Database

    An interactive Java applet lets you select, multiply and visualize unit cell data of various minerals on Crystal Structures




    Footnotes

    1 The m-plane is a crystallographic plane parallel to one of the m-faces that form the hexagonal prism of the crystal.

    2 It is sufficient to take three motifs to form the inner ring of SiO4 tetrahedra around central channel.

    3 I will not explain the meaning of the symbols as that is beyond the scope of this topic and I must assume a basic understanding of DNA structure. Check out the link to the Wikipedia article on DNA.

    4 You will note that there are actually six directions, but they lie on only three axes, and the two directions on each axis are equivalent.

    5 The fact that there are three axes is a special property of hexagonal patterns, it can only be found in structures in which the axes meet at an angle of 60°.

    6 Hexagons can cover a plane completely to form a honeycomb pattern, but their edges will not join to form the straight axes of a coordinate system.

    7 This is just a matter of convenience for me, as the basic data set that has been used to render all figures on this page uses the coordinates of the left unit cell model.

    8 As I have already mentioned above, the fact that the unit cell is face-centered is the result of an arbitrary decision on its vertical position.

    Printer Friendly Version

    Copyright © 2005-2016, A.C. A k h a v a n

    Impressum    -    Source: http://www.quartzpage.de/gen_struct.html