last modified: Sunday, 04-Sep-2011 22:12:57 CEST
Document status: usable, incomplete, some images missing
When it comes to physical properties, quartz is pretty much an average mineral and does not show outstanding or extreme properties. Nevertheless quartz is a technically important material that is valued for the combination of certain electrical or optical properties with a great physical strength and chemical resistance.
In this chapter I will not simply count off physical properties of quartz and be done with it. Instead, I will try to explain what is behind the properties. I will start with a discussion of what is the base of its physical behavior (albeit it is not a physical measure as such): its crystalline structure.
Anisotropy and Crystal Structure
Minerals have a number of unique physical properties that are very different from that of other solid substances, like plastic, wood, concrete, bone, or glass. For example, some may change color depending on the angle of view, others may get electrically charged when put under stress, may only part easily in one direction, or may be scratched more easily on one crystal face than another.The cause of this odd behavior is the crystalline nature of minerals. In a crystal the atoms are arranged in a regular and periodic manner that is specific for each mineral. The geometry of this arrangement of atoms is not only reflected in the symmetry properties of a crystal, but also in the isotropy or anisotropy of its physical properties. A substance that reacts differently depending on the direction of an external force[1] is called anisotropic. Different minerals are anisotropic to various degrees, and many of the physical properties of quartz show anisotropy[2].
Not all physical properties can show anisotropy, others can, but don't always; the following table gives an overview for the most important physical properties.
| Never anisotropic |
Specific Density Melting Point Boiling Point Heat Capacity Streak |
| Can be anisotropic, but is isotropic in quartz |
Color (colored varieties may show dichroism) Diaphaneity Luster Fracture Dispersion (?) |
| Anisotropic in quartz |
Refraction Birefringence Optical Activity Hardness Cleavage Thermal Conductivity Thermal Expansion Coefficient Piezoelectricity Relative Permittivity |
The next 4 figures shall demonstrate schematically how the symmetry of the internal structure relates to anisotropy. The atoms of different elements are symbolized by circles of different size and color.

|

|

|
|
Note: it is not possible to deduce the anisotropy of a specific physical property from the external crystal form. The example "crystals" in the drawings presented above would all qualify as cubic or isometric crystals, yet they would show different anisotropic behavior. In fact, cubic minerals are all optically isotropic, but some are electrically anisotropic. What counts is the internal symmetry - crystals that lack a center of symmetry or mirror symmetry in their structure are apt to show some type of anisotropy.
Although quartz has a very simple chemical formula, its internal molecular structure is complex and lacks mirror symmetry - an ideally grown quartz crystal is either left or right handed. In nature, many crystals are twinned, that is composed of subindividuals whose internal structures are oriented differently and intergrown in a law-like manner. Untwinned natural quartz crystals were once commercially mined, because their anisotropic electrical and optical behavior has important technical applications. Nowadays untwinned quartz crystals are artificially grown on an industrial scale.
Optical Properties
To most people, rock crystals look very much like cut and polished glass. However, rock crystals - and many other crystalline substances - have a number of optical properties that differ very much from those of glass. To understand what is so special about crystals one should briefly recall some of the things from a physics course in school.
|
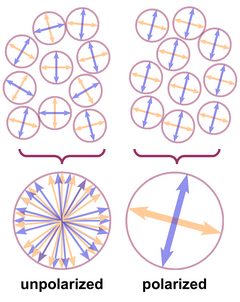
The wave planes of light rays emitted from a lamp or from the sun are oriented randomly, and such light is called unpolarized, as shown in Fig. 2. This is symbolized by a bunch of randomly oriented arrows (field vectors) in a circle. If all electric and all magnetic field vectors show the same respective orientation, the light is polarized.
The atoms in a crystal - and in any solid substance - are not just regularly stacked "billiard balls", they are electrically charged and a ray of light that enters a crystal enters a regular mesh of vibrating electric charges and complex electric fields. Its own electric and magnetic fields interact with the fields already present in the crystal.
Color, Streak, Luster, Diaphaneity
Pure quartz is clear and colorless. It is not only transparent for visible light but also for long wave ultraviolet radiation. This is also true for fused quartz (amorphous quartz glass), which is used as a replacement for ordinary glass in ultraviolet lamps for that reason.Colored varieties of quartz owe their color to trace elements built into the crystal lattice[5] or to the inclusion of other, usually colored minerals.
The streak of macrocrystalline quartz varieties is colorless or white, even a black morion has a white streak. This also holds for cryptocrystalline varieties, unless the amount of embedded colored impurities is very high; then the streak is slightly colored. For example, the streak of an opaque dark red jasper can have a bright salmon red color.
Quartz has a vitreous, "glassy" luster. Fracture surfaces sometimes show a greasy or fatty luster. Cryptocrystalline quartz has a waxy luster, on fractures it is dull to waxy. The quality of the luster is related to the surface structure and to the refractive index, so even an opaque jasper or flint has a vitreous luster when polished.
Refraction and Birefringence
|
Like many other minerals, quartz shows a very interesting property called birefringence or double refraction. This phenomenon is well known from calcite: when a clear calcite rhombohedron is put on a newspaper, one can see a double image of the letters. A single ray of light is split up into two rays while it passes the rhombohedron. Birefringence is present in many crystallographically non-isometric (non-cubic) materials and is absent from amorphous materials, isometric (cubic) minerals, and liquids.

|
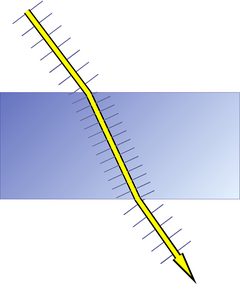
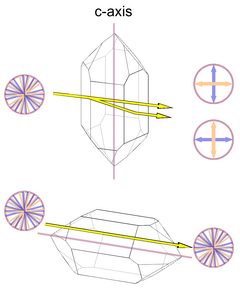
Figure 4 shows refraction and polarization in three cases.
1. Top: In an isotropic substance, like glass, a liquid, a gas, or a mineral of the cubic crystal system, the polarization of light is not changed;
2. Center: A light beam consisting of randomly polarized light waves will be split into 2 beams of perpendicular polarization;
3. Bottom: A single light wave will be decomposed into 2 waves with a polarization perpendicular to each other.
The two rays are called ordinary and extraordinary rays, and the corresponding refractive indices are symbolized as no and ne. The value of birefringence is determined by subtracting no from ne, and can be positive or negative. The next table lists a few refractive indices for comparison. Diamond is crystalline, but cubic and thus isotropic, and does not show birefringence.
| Ordinary Ray no | Extraordinary Ray ne | Birefringence | |
| Water | 1.3299 | - | - |
| Glass | ca. 1.5 | - | - |
| Silica Glass | 1.45886 | - | - |
| Quartz | 1.54422 | 1.55332 | + 0.00910 |
| Calcite | 1.65838 | 1.48643 | - 0.17195 |
| Diamond | 2.4173 | - | - |
The birefringence is positive and very low in quartz, and is very difficult to observe directly. It is still big enough to be a drawback when using quartz (as opposed to silica glass, or cubic and isotropic fluorite) in ordinary optical apparatus.
|
The optical axis in quartz corresponds to the c-axis of the unit cell, so there is no birefringence when light passes the crystal from tip to tip. The maximum birefringence occurs when the light passes perpendicular to the optical axis (Fig.5, with the amount of birefringence greatly exaggerated). Light that passes the crystal along the c-axis will also not be split into two rays of opposite polarization.
Interestingly, Frondel (1962) reports that Fresnel (1831) found quartz to be slightly birefringent along the c-axis, the value given is 0.000071. This value is below the detection limits of most methods, so by any practical definition quartz still lacks birefringence along the c-axis. I have no further reference of the value given by Frondel, and it is not clear if this value was determined by Fresnel or by Frondel.
Dispersion
Because light of a short wavelength (e.g. blue) is refracted more than long wavelength light (e.g. red), white light passing a transparent medium will be split up into the colored components of its spectrum, a phenomenon called dispersion. Dispersion of light in water drops is what is causing the rainbow, and is the reason for the "fire" of a brilliant cut diamond.The coefficient of dispersion is a measure of how much the refractive index of a substance depends on the wavelength of the light. Quartz has a low coefficient of dispersion and thus cannot be used as a diamond imitation. In optical apparatus dispersion needs to be kept as low as possible, for example to avoid chromatic aberrations (known as "purple fringing") of a camera lens. Note that dispersion is not a phenomenon confined to anisotropic crystals, it can be observed in isotropic crystals and non-crystalline substances as well, for example ordinary glass, or water (causing the rainbow). Its value can nevertheless be anisotropic.
The following table of refractive indices for both the ordinary no and the extraordinary ray ne (quartz is birefringent) lists values given by ->Bauer, with colors given instead of wavelengths.
| Red | Yellow | Green | Blue | Violet | |
| no | 1.5409 | 1.5442 | 1.5471 | 1.5497 | 1.5582 |
| ne | 1.5499 | 1.5533 | 1.5563 | 1.5589 | 1.5677 |
Dichroism
A transparent substance whose color depends on the direction of the light passing through it is called pleochroic. If it changes between two colors, which correspond to two crystallographic axes, it is called dichroic. Pure quartz is colorless and cannot be dichroic, but some of its colored varieties show a weak dichroism:
natural citrine[7]: yellow/bright yellow
smoky quartz: yellow-brown/red-brown
amethyst: gray- or blue-violet/red-violet
ametrine: only the amethyst sectors: gray- or blue-violet/red-violet
prasiolite: yellow-green/blue-green
The problem in observing the color change is i) that the effect is very weak and ii) that one needs a clear double terminated crystal to compare the color of light passing parallel to the c-axis and perpendicular to it. With the naked eye it can only be observed in minerals in which the effect is very strong (cordierite is the standard example).
To demonstrate dichroism in quartz, we need to use a polarizing filter. We could simply use a circular or linear polarizer lens for a camera. But if you sit in front of a LCD or TFT computer screen (a flat screen and not a CRT monitor) or a laptop, you don't even need that, because these types of computer screens emit polarized light. First choose a white background for the screen, then take a clear but dark smoky quartz crystal and hold it before the LCD display with its crystal tip pointing upwards. Slowly rotate the crystal, for example, clockwise so the tip points to the right and then downward, around, and up again and notice how the color changes gradually between a darker yellow and a brighter red tone. If you take a polarizer lens, you would rotate the lens before or behind the crystal, whatever is more convenient. This also works with natural citrine[7], although only the color intensity variations are obvious, the color tone doesn't seem to change. Amethyst shows very weak color variations that are difficult to observe unless you have a well-formed prismatic crystal.
Optical Activity
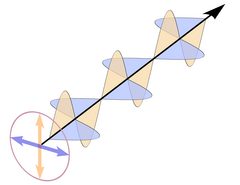
Substances that rotate the polarization plane of light waves passing through them are called optically active. This does not involve the polarization of unpolarized light, as described under "birefringence" - instead, polarization planes that are oriented differently will all be rotated by the same amount (schematically shown in Fig. 6, identical angles of rotation are indicated by the turquoise sectors). This phenomenon has actually been discovered in quartz (by Dominique Arago), but is now better known from glucose sugar. Only enantiomorphous substances, those that lack mirror symmetry, can show optical activity. In sugar the individual molecules are enantiomorphous, so the effect can even be observed in a watery solution of sugar. In quartz the crystal structure shows enantiomorphy as a whole.

Fig.6: Optical Activity
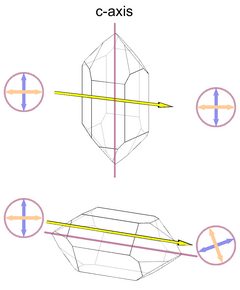
|
The direction of the rotation depends on the symmetry of the crystal. There is right and left quartz, reflecting the orientation of helicoidal or corkscrew-like chains of SiO4 units in the quartz crystal structure. These helicoidal SiO4 -chains are oriented parallel to the c axis, so they run from tip to tip of the crystal. Interestingly, the polarization planes rotate in the same direction, either left or right, as the SiO4 -chains.
So in an untwinned crystal these SiO4-chains all turn left- or rightward. But in a Brazil law twin left and right quartz structures are intergrown and the effects of left and right quartz structures on the light ray will cancel each other out, at least partially. Brazil twins are accordingly called optical twins. In Dauphiné twins left and left or right and right structures are intergrown, and these crystals are as optically active as untwinned crystals.
To practically observe this effect one needs to use monochromatic polarized light, light of a definite color in which all light waves oscillate in the same plane. The reason for using monochromatic light is that the amount of rotation depends on the wavelength of the passing light. It is much less for infrared than for ultraviolet light. As you can see in the following table, the effect is quite strong: even plates of just a few centimeters in thickness will rotate the polarization plane of visible light (ca. 300-700nm) passing through them several times.
| λ [nm] | 275 | 373 | 436 | 486 | 509 | 589 | 656 | 1040 | 1771 | 2140 |
| γ / mm | 121.1° | 58.86° | 41.55° | 32.67° | 29.72° | 21.73° | 17.32° | 6.69° | 2.28° | 1.55° |
| Optical Properties | |
|---|---|
| Color | colorless if pure, otherwise any color |
| Streak | none / white |
| Luster | vitreous Cryptocrystalline: waxy to dull, vitreous if polished |
| Diaphaneity | transparent to opaque |
| Refractive Indices | no=1.54422 ne=1.55332 Cryptocrystalline: n = approximately 1.54 |
| Birefringence | uniaxial positive: ne-no = +0.00910 lower in cryptocrystalline quartz |
| Pleochroism / Dichroism | none if pure, weak dichroism is observed in smoky quartz, amethyst, and certain citrines |
| Coefficient of Dispersion | θo=0.00779 θe=0.00807 |
| Fluorescence | none if pure |
| Optical Activity | parallel to c-axis |
Electrical Properties
Quartz is an electrical insulator, as there are no freely movable electrons in its crystal structure like in metals. Nevertheless quartz shows an interesting behavior when exposed to electric fields.Piezoelectricity
This is the technically most important property of quartz and it has numerous applications. Piezoelectricity was discovered by Jacques and Pierre Curie in 1880, during studies on quartz crystals. When piezoelectric materials are mechanically deformed, their surfaces get electrically charged. The reverse is also true: the same materials will get deformed when put into an electric field. Piezoelectricity is once again an anisotropic property that can be found in certain types of crystals, but not in amorphous substances, and that is dependent on the direction of forces.

|
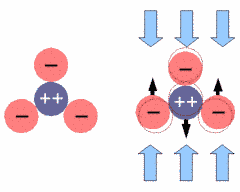
|
Under mechanical stress the atoms of the tetrahedron get displaced with respect to their former position (indicated by a thin red outline). The positively charged silicon is pushed away from its central position and the whole structure gets electrically polarized. If one reverses the direction of the forces, the silicon will be pulled upwards, resulting in an opposite polarization.
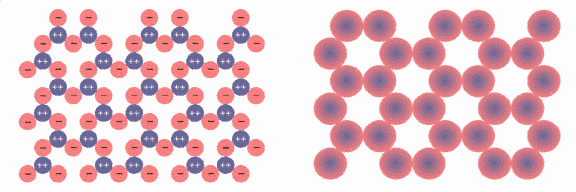
Fig.10: Simplified Model of a SiO2 Network
In a quartz crystal, the SiO4 tetrahedra form a network in which the units have a defined position and orientation. Figure 10 shows a simplified model of a SiO2 network, with all SiO4 units being oriented the same way. In reality, the situation is far more complex: not the individual tetrahedra are oriented the same way, but groups of tetrahedra; the principle still holds, however.
We can view each SiO4 unit as a sphere that has a positively charged core (dark blue) and a negatively charged shell (red), as depicted in Fig.10 to the right.
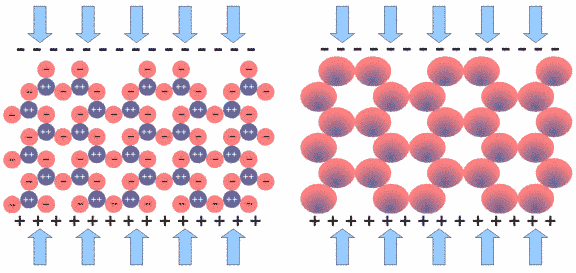
Fig.11: SiO2 Network under Mechanical Stress
If we put the crystal under mechanical stress, as shown in Fig.11, all tetrahedra are effected, with their central silicon atom pushed downwards. All SiO4 units are polarized in the same way, in this case being more negative on the top and more positive on the bottom, as depicted in Fig.11 to the right. The voltage built up in each SiO4 unit is very small, but since millions of them line up in the crystal structure, their voltage adds up to a measurable amount. This is very similar to lining up batteries in a torch. In fact, one can measure several thousand volts on the crystal surface.
As I said, the molecular structure of quartz is much more complex than the structure shown in the figures, and of course its behavior under stress is more complex as well. The direction of mechanical forces that is most effective in polarizing a quartz crystal is parallel to its a-axes, while forces parallel to the c-axis show no effect. There are 3 a-axes in quartz, however, only 2 different surface charge distribution patterns.
HISTORY OF PIEZOELECTRICITY, Piezo Systems, Inc.
http://www.piezo.com/tech4history.html
PIEZO TERMINOLOGY, Piezo Systems, Inc.
http://www.piezo.com/tech1terms.html
http://www.physikinstrumente.com/en/products/prdetail.php?VID=&sortnr=400600.00
http://en.wikipedia.org/wiki/Piezoelectricity
To be completed...
Pyroelectricity, a closely related phenomenon, was discovered first by T. Epinus in 1762 on tourmalines. Tourmaline crystals had been used for a long time by traders from Holland to remove ash from their tobacco pipes (called "Aschentrekker"). When the crystals heat up in the pipe they polarize electrically and both crystal ends develop opposite charges. The same seems to happen in quartz, but as in the piezoelectrical effect, the polarization axis is parallel to the a axis, not the c axis as in tourmaline. However, the pyroelectricity observed in quartz is simply a side effect of its piezoelectricity and the fact that its thermal expansion coefficient is anisotropic (see under "Mechanical Properties"): a crystal that is heated up quickly will suffer the same stress and strain as if mechanical pressure was applied. If you heat up a quartz crystal slowly and evenly, it will not show pyroelectricity.
Relative Permittivity or Dielectric Number
Permittivity is a measure that characterizes the changes of the electric field density E when a substance is placed in an electric field. Its unit is the dielectric number εr (sometimes called dielectric constant). The dielectric number can be used to roughly estimate how much a substance gets electrically polarized in an electrical field: the values for benzene, ethanol, methanol, and water are 2.28, 25.1, 33.5, and 81, respectively, as these liquids consist of increasingly polar molecules that re-orient themselves in an electric field.The value of εr for quartz is between 4.69 parallel to the a-axis and 5.06 parallel to the c-axis, it is yet another anisotropic physical property. The dielectric number of quartz is not much different from that of glass (3-15), wood (3-5), or plastic (2-5). Because it is chemically inert and mechanically very enduring, it is nevertheless an attractive dielectric, and is used, for example, to separate electrical circuit elements inside computer chips.
Magnetic Properties
Quartz is diamagnetic. It will be forced out of a magnetic field, but this force is extremely small.
Mechanical Properties
Hardness
The first thing that comes to mind when thinking about mechanical properties of quartz is its great hardness. Quartz will easily scratch glass and most metals. Of course, there are variations of hardness depending on which crystal faces and edges you scratch.Hardness can be defined and measured in many ways. The most common unit is the value on the Mohs scale. Here a mineral's hardness is estimated by comparing it with the hardness of 10 reference minerals that have been given the following Mohs hardness values:
A mineral with a higher Mohs hardness value can scratch one of equal or lower value. So minerals of equal hardness do also scratch each other. Quartz by definition has a Mohs hardness of 7, cryptocrystalline quartz is a bit softer and given a value of 6.5-7. Note that this is a relative scale, and that the steps on the scale are not equal (so feldspar is not twice as hard as calcite). This scale is in use because it is easy to memorize and because a comparative test is easy to do.
There are several methods to measure hardness directly to determine an absolute value. The most important is the Vickers indentation hardness, which reflects the size of a dent that is caused by a diamond tip pressed onto the material with a force of 1 Newton for about 15 seconds. The value given for quartz is 1181 kg/mm2, but it is larger on the m prism faces (1260 kg/mm2) than on the basal plane c (1103 kg/mm2), an obvious anisotropy.
The Rosival grinding hardness is a relative measure, like the Mohs scale, but based on an exact procedure: The amount of material removed from the probe by grinding is determined and compared with other materials. Quartz is used as a reference and is given a value of 100.
When the results obtained by both methods (and others not mentioned here) are compared with the Mohs scale, the order is preserved, but the Mohs scale turns out to be roughly logarithmic - the difference in hardness between corundum and topaz is much larger than between fluorite and calcite.
In a similar approach, using a sandblast machine, Milligan, 1936, has measured the hardness of various crystal faces and found the hardness of the faces to decrease in the order:
1. positive rhombohedron (r-face)
2. positive prism (m-face)
3. basal plane (c-face)
4. negative prism (a face)
5. negative rhombohedron (z-face).
Note: Quartz will scratch quartz! I once saw a posting in a newsgroup in the Internet: Someone had bought an artefact supposedly made of rock crystal and had paid a good price for it, but he was suspecting it might actually be glass and was asking for an advice. Someone else told him he should just get a piece of real quartz and try to scratch it, if it was glass, it would be scratched - a very bad advice. Should the artefact in fact be quartz, it would still be scratched and the poor owner would have damaged his valuable piece. He might even dispose of it in anger as he falsely assumes to be the victim of a fraud, or sue the innocent dealer.
It would be a bit better to do it the other way: Take a piece of window glass and carefully[8] try to scratch the artefact. Clean the surface of the fine grains of glass that probably come off during the scratching process and use a magnifying lens to inspect the surface. If it gets scratched, it is very likely not quartz. This could of course still damage the artefact.
There are better methods to non-destructively determine the nature of an artefact. If in doubt, consult a nearby jeweler who should have instruments to determine its optical properties (which should be very different from that of glass).
Fracture and Cleavage
Pure macrocrystalline quartz shows a conchoidal fracture that is very similar to that of glass. Massive quartz has an uneven fracture, and the same can be observed at the milky base of many crystals. In many cases the fracture is an irregular mixture of both uneven and conchoidal structures. As already mentioned, the luster of these fractures is vitreous/glassy to fatty. Cryptocrystalline varieties fractures tend to be simply conchoidal, but more even. The luster of cryptocrystalline fractures is dull to waxy.
Homogeneously formed quartz crystals don't show an obvious preferred direction of parting or cleavage. They seem to break in a quite unpredictable manner, a fact that makes the removal of quartz crystals from vugs and pockets so difficult. Great care must be taken if a crystal is to be removed in one piece. Aggregates of quartz crystals sometimes behave differently and it is possible to work out individual crystals because they have shrunk with falling temperatures and the crystals are not as intimately intergrown as they were during their formation.
However, when the behavior of a great number crystals is measured and analysed with appropriate statistical methods, one observes a tendency of crystals to break parallel to the rhombohedral faces, in particular the r-face belonging to the positive rhombohedron. A review of earlier papers is given in Bloss and Gibbs 1963, in addition to own, quantitative measurements, in which they found the tendency of cleavage to be greatest parallel to the rhombohedral faces (r and z were not distinguished), less so parallel to for prism faces (m and a were not distinguished), and to a much smaller degree (but still detectable) parallel to the basal plane (c). They suggest differences in the density of the atomic lattice on the crystal faces as the cause for the differences, as the number of bonds per area is lowest along the r-face. In a more recent study, Flörke et al., 1981, found the rhombohedral cleavage to predominantly occur along r-faces, and much less along the z-faces. The cleavage was observed as numerous straight cracks running parallel to crystallographic planes in tectonically stressed quartz veins in Madagascar. They found cleavage in most cases to be associated with polysynthetical twinning according the Brazil law, resulting in crystals that are made of alternating layers of left- and right-handed crystal structure, and a cleavage parallel to the twin boundaries (unfortunately, Bloss and Gibbs did not check for twinning or lamellar structure of the crystals).
Tenacity
Quartz is quite brittle, and despite its hardness it can be much easier for a rockhound to work his way through a quartz vein than through adjacent softer but malleable rock that will simply absorb the strikes of the hammer. Cryptocrystalline quartz varieties are a bit softer but also tougher and less brittle, one of the reasons why agate was once used for laboratory equipment.Quartz is elastic to some degree, of course not like a rubber band, but enough to be used in oscillators and as a membrane in ultrasonic loudspeakers.
Other Properties
Many people think that quartz has a very low thermal expansion coefficient and can easily withstand quick changes in temperature. This is not true. Silica glass (quartz glass a.k.a. fused quartz) has a thermal expansion coefficient about 1/18th of that of ordinary glass; a red hot silica glass rod can be dipped into water without cracking. Lenses made of silica glass and fixed in a rigid apparatus do not get distorted by changes in temperature.On the other hand, quartz has a thermal expansion coefficient that is only slightly lower than that of ordinary glass and as you know no one in his right mind will serve hot tea in a wine glass. The thermal expansion coefficient is also anisotropic, and is smaller parallel to the c axis: if you heat up a crystal, it will get more elongated. For a single, isolated crystal this doesn't matter too much, but in a crystal group the individuals will expand differently in different directions, which might induce considerable stress.
The thermal conductivity of quartz is higher than that of glass, so overall it can take a bit more [9], but it's still better to handle quartz crystals with care. In general, quartz feels colder than glass. The thermal conductivity is anisotropic and higher parallel to the c-axis.
Further Information, Literature, Links
A table of quartz properties can be found at mindat.org:http://www.mindat.org/min-3337.html
Another table at www.webmineral.com:
http://www.webmineral.com/data/Quartz.shtml
If you can read Italian, you should have a look at Marino Bignami's very nice introduction to birefringence and polarization on quartz, a section of his remarkable www.faden.it page.
Footnotes
1 The term force in this context also includes electromagnetic fields, like light passing through a crystal, or the exchange of energy, e.g. the flow of heat.2 There are physical properties whose values by definition are independent of direction, and which have so called scalar units, for example the specific weight, temperature, or melting point of a substance. If a physical property or value includes information about a direction or is dependent on it, its unit is a vector, for example speed, acceleration, or electrical conductivity.
3 That would be 3 principal axes in a three-dimensional structure, of course, but the figure is only two-dimensional. These principal axes correspond to the three crystallographic axes of a unit cell.
4 Light is neither a particle nor a wave, and describing it as a wave only means that we describe one aspect of its behavior and disregard those properties that make it appear as a particle.
5 Usually in conjunction with high energy radiation from radioactive sources which causes the generation of color centers. With the exception of certain citrines, the concentration of trace elements built in is too low to cause visible changes in color.
6 The speed of light is always the same, no matter what medium it travels in. It is quite difficult to understand what effectively slows it down. If you are interested, Chapter 33 in Volume I of ->The Feynman Lectures on Physics discusses the matter thoroughly.
7 Burnt citrine (made from amethyst) is not dichroic.
8 If you apply too much force, the piece might still get damaged, as quartz is very brittle and might eventually "give in". People who call themselves flintknappers work on flint with copper and wooden tools, although wood and copper are much softer than flint.
9 If a substance has a low thermal conductivity its surface will expand quickly when exposed to a heat source while its interior remains cold for some time. That introduces a lot of strain and can cause the material to crack. The higher the thermal expansion coefficient and the lower the thermal conductivity, the more sensitive the substance is to quick changes in temperature. That′s why a knowledgeable rockhound will never touch clear sulfur crystals: their thermal conductivity is very low and they will get dull because of tiny cracks that form on their surface when you frequently touch them with your warm fingers.
 Printer Friendly Version
Printer Friendly VersionCopyright © 2005-2013, A.C. A k h a v a n
Impressum - Source: http://www.quartzpage.de/gen_phys.html