Appendix - Ternary Diagrams

 

last modified: Tuesday, 26-May-2009 03:44:40 CEST

Document status: complete

Ternary Diagrams

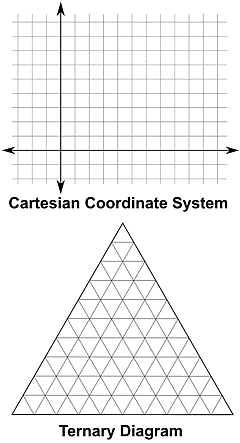
ternary_1.png
Fig.1.1

A ternary diagram is simply a triangular coordinate system in which the 3 edges correspond to the axes. Figure 1.1 shows a sketch of the familiar Cartesian coordinate system and of a ternary diagram.

Except for the obvious difference in shape, there are three important differences:

  • A two-dimensional Cartesian coordinate system is based on 2 axes, and each point is defined by 2 values (for example x and y). In a ternary diagram there are 3 axes and each point is defined by 3 values.
  • A Cartesian coordinate system may be "open" and have axes of infinite length; a ternary diagram is always "closed" and has finite axes.
  • A ternary diagram is used to plot dependent variables that always add up to a fixed value.

    • Ternary diagrams are often used to visualize the composition of rocks or minerals, because the percentual values are dependent variables: the "dependence" between the components is the fact that they must add up to 100%, so if we have a rock made of 2 minerals A and B, and we know the value of one variable (for example, 20% of mineral A), we can calculate the other (100% - 20% = 80% of mineral B).


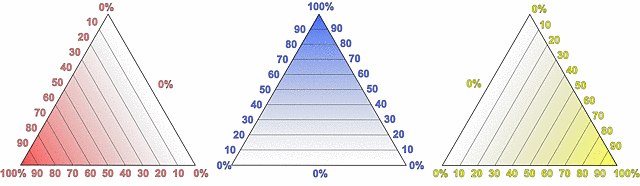
    ternary_3.png

    Fig.1.2

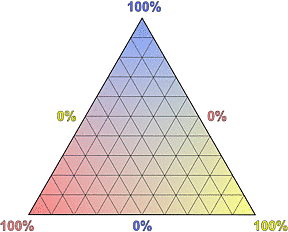
    ternary_3.png

    Fig.1.3


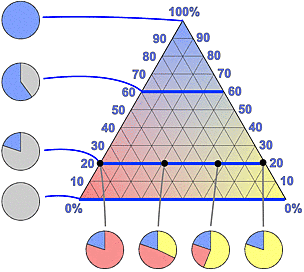
    ternary_4.png
    Fig.1.4

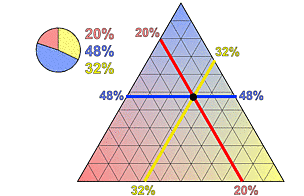
    ternary_5.png
    Fig.1.5

    In a ternary diagram of the composition of a rock, the 3 edges of the triangle represent axes of the concentration of 3 components. How the composition changes within the triangle and how the axes are drawn is shown independently for each component, symbolized by red, blue and yellow colors in various intensities corresponding to the relative concentration, in Fig.1.2. The corners represent the extreme cases in which only one component is present.

    Figure 1.3 shows the final ternary diagram, the combination of the three triangles from Fig.1.2. Note that now each edge of the triangle represents 2 axes with opposite directions for 2 components, going from 0% to 100% for one component and from 100% to 0% for the other. Because it would simply look confusing, the complete annotation of the axes is usually not shown.

    Figure 1.4 explains how the diagram is read. To the left we see four pie charts showing different contents of the "blue component". A content of 100% corresponds to the top corner, but a lower content, like 0%, 20% or 60%, is always represented by a line in the triangle.

    The relative concentrations of the other components (red and yellow) change along these lines, as shown for 4 positions along the 20%-line. The corresponding pie charts with all components are shown at the bottom.

    Finally, Fig.1.5 demonstrates how to plot the composition of a rock into a ternary diagram. The position is simply the intersection of the lines that correspond to the 3 percentual values.


    Printer Friendly Version

    Copyright © 2005-2013, A.C. A k h a v a n

    Impressum    -    Source: http://www.quartzpage.de/app_td.html